A modern blast furnace produces up to 5 mio tons of iron / year and ca be operated continuously for up to 10 yeas before shutdown.
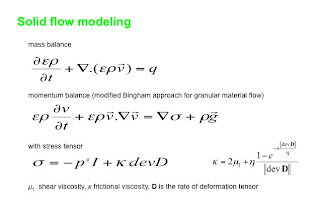
To control the process you need to model various phenomena, like flows of iron ore and coke, liquid due to melting, gas - from bottom to top, energy - heat conduction and convection, mass and chemical reactions.
As the iron ore sinks it is indirectly reduced: Fe2O3 --> Fe3O4 --> FeO --> Fe.
The typical sitze of the problem: 40.000 spatial unknowns per scalar component of 20-30 unknown functions (temperature, max concentrations, velocities).
Computing time on a PC: about 4 hours for a real-time day. Not bad for faster-time-to-insight and decisions.
Again, what has this to do with computational finance?
The equations are similar (convection-reaction-diffusion) and consequently we have transferred the optimized PDE and Fourier based solvers to finance and organize them orthogonally to instruments and models - enabling model validation ...
Our numerical repertoire for valuations embraces Adaptive Integration (a asymptotic math method), Finite Elements with Streamline Diffusion, Fourier-based methods (linking with cos methods), all transferred from complex technical systems, and tuned and modified Monte Carlo schemes (including least square and Longstaff Schwartz).
Embedded in a center focussing on inverse problems research, our calibration engines are blazingly fast and robust.
Stable and robust cross-sectoral mathematics really matters.
